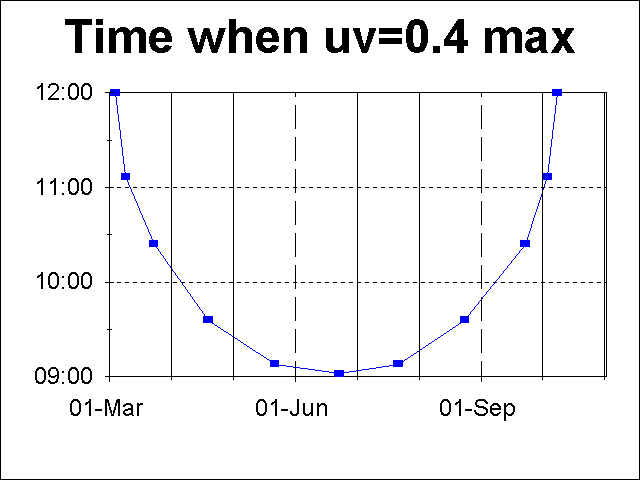
Fig. 4. Time of day in the morning (local time) at which ultraviolet light from sun equals 0.4 times the maximum at noon on the summer solstice, ~21 June.
Here's the quick answer for a latitude of 33.3° versus calendar date:

Remember that the afternoon time is (noon) + (noon - morning time).
Here's the long answer, including how to compute the same curve for any latitude:
Remember the constant advice we get in the summer to stay out of the sun between 9 am and 3 pm? Over the Christmas vacation of 1995, I got interested in quantifying that advice versus date after reading a Sky & Telescope article that gave the dependence of uv on sun angle, especially to find out what the corresponding times were in winter.
The following table gives the results for the latitude of Fallbrook, CA, 33.3°.
| UV Intensity (fraction of maximum) | dates | ||||||
|---|---|---|---|---|---|---|---|
| sun declination | sun angle at noon | 0.5 | 0.4 | 0.3 | 0.2 | 0.1 | |
| 23 | 10.3 | 9.37 | 9.03 | 8.67 | 8.3 | 7.8 | 21 Jun |
| 20 | 13.3 | 9.5 | 9.13 | 8.77 | 8.4 | 7.87 | 21 May, 21 Jul |
| 10 | 23.3 | 10 | 9.6 | 9.23 | 8.8 | 8.3 | 19 April, 24 Aug |
| 0 | 33.3 | 11.07 | 10.4 | 9.87 | 9.4 | 8.8 | 21 March, 21 Sep |
| -10 | 43.3 | 11.2 | 10 | 9.53 | 24 Feb, 18 Oct | ||
| -20 | 53.3 | 10.87 | 21 Nov, 21 Jan | ||||
| -23 | 56.3 | 12 | 21 Dec | ||||
The first column is the declination of the sun, and the right hand column gives the approximate dates at which the sun reaches that declination. The times are the local (~standard) times of the time in the morning at which the uv from the sun reaches the given percentage of maximum on 21 June. The afternoon time that corresponds to the same level of uv is 24 - morning time, using 24 hour time, or 12 - morning time in p.m. time.
The conventional advice implies that one should stay out of the sun anytime the intensity is greater than 0.4 of maximum. That would imply staying out of the sun between:
For those interested, it turns out the uv drops off much more steeply than just the path length through the atmosphere, presumably because the atmosphere is optically thick to uv. Since the received uv radiation dies out quickly at earlier and later times, there is not much benefit to picking a number smaller than 0.4 of maximum uv. In other words, the total received uv radiation is relatively small up to the time when the intensity hits 0.3 or 0.4 of maximum uv. However, one will receive quite a bit more total uv radiation by staying out even a bit later than those times, since the uv quickly increases to the maximum.
If anyone wants a Quattro Pro or Excel spreadsheet that does the above calculation, let me know. It can easily be customized to give the results for any latitude, although the spreadsheet is not highly automated, requiring the user to construct the table above by varying parameters.
A quicker way to get the results for most other latitudes is to use the column giving the sun angle at noon. The sun angle at noon is your latitude minus the solar declination for latitudes above 23°. (Take the absolute value of the difference for lower latitudes.) If your latitude is 50°, for example, at the summer solstice on 21 June, the sun angle at noon would be 50 - 23 = 27°. This is a bit less than halfway between the sun angles of 23.3 and 33.3 in the table. Hence the "9 am to 3 pm" advice translates to "10 am to 2 pm" for you.
Although the table is incomplete for smaller sun angles, at a sun angle of 0° there is only a 4% increase in the amount of uv radiation at noon. Hence the times are little changed. San Diego County is already nearly the worst case for maximum uv, having a sun angle of 33° - 23° = 10° at noon on June 21. The absolute maximum would of course be at a latitude of +23°. The equator receives less uv radiation on June 21, having a sun angle at noon of 0° - 23° = -23°, equivalent to Fallbrook's radiation in April and August! (The received radiation is the same for positive or negative sun angles.)
Finally, note that i don't recommend any amount of exposure to the sun at all. If you have been exposed to a fair amount of sun, typical for those over 40 years old, take a look at the skin on your face and hands and compare it to places that have received very little sun, like perhaps your stomach or buttocks. The difference in the skin's appearance is solely due to sun damage.
There were absolutely striking facial pictures in Science News of September 24, 1988 of a 91 year-old Zen monk who had virtually never been exposed to sun and that of a 62 year-old Native American farmer who had been constantly in the sun. From the appearance of the faces, you would have guessed that the monk was at least 30 years younger than the farmer, instead of the other way around. (Science News 134:200.)
Thanks to Keith Davies for a good question about the 0.4 maximum that improved this page. If anyone knows the references to the Sky & Telescope article, or the Science News article, please let me know.
Go To:
Copyright © 1996-1999 by Tom Chester.
Permission is freely granted to reproduce any or all of this page as long as credit is given to me at this source:
http://sd.znet.com/~schester/calculations/sun_avoidance_times.html
Comments and feedback: Tom Chester
Last update: 26 May 2004.